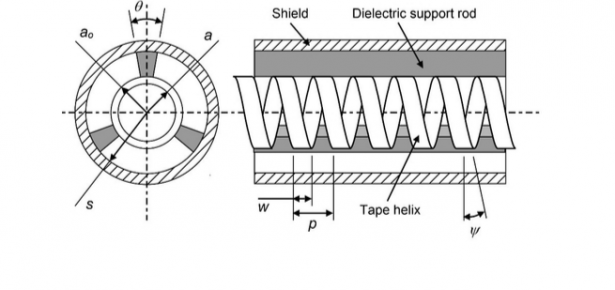
An essential component in the helix travelling-wave tubes (TWTs) used, among other things, for satellite communications and electronic counter-measures, is the helix slow-wave structure. This structure comprises a helically-wound wire that is located within a concentric metal cylinder by three dielectric support rods (see the figure below). The structure is essentially a coaxial transmission line with a helical inner conductor. The electrical properties of the structure (phase velocity and coupling impedance) can be calculated using computational electromagnetics software. However, this is too slow for use in a computer algorithm for optimising the performance of a TWT.
Many papers based on electromagnetic field modelling have been published but the goal of good agreement with the results of experimental measurements has been elusive. A critical summary of the literature can be found in Chapter 4 of my book Microwave and RF Vacuum Electronic Power Sources (Cambridge, 2018) pp.159-162. The difficulty is that it is necessary to make simplifying assumptions to make the problem tractable. The earliest papers analysed the sheath helix slow-wave structure in which the helix was represented by a cylinder in which the current flow was restricted to flow in the helical direction. This model gives a useful qualitative understanding of the properties of these structures but does not provide accurate results (see pp.154-159). An important development was the recognition that the equations of the sheath helix model could be recast as those of a TEM line whose shunt capacitance and series impedance depended on frequency through the propagation constant.
I became interested in this problem and took the opportunity of a spell as a Visiting Scientist at the Central Electronics Engineering Research Institute in Pilani in India in 1992 to try to develop an equivalent circuit model in which the component values were constant. The model represented the helix by a set of equally-spaced rings which were coupled to one another by mutual capacitances and inductances. I found that the model could be fitted quite well to experimental data if each ring was coupled to the two nearest rings on either side. I subsequently made a number of attempts to calculate the coefficients of capacitance and inductance with limited success as described in papers published in 1994, 1995, and 1997. My attention turned to other things and I did not return to the problem until working on my book during 2013. A review of the literature on planar coupled lines showed that the same approach could be used there. The transverse dimensions of such a line are small compared with the free-space wavelength so the coefficients of capacitance can be computed using numerical solutions of Laplace’s equation implemented using a spreadsheet (see. pp.145-148 and Worksheet WS 4.1 which is available for free download in both Mathcad and pdf formats from the resources tab of the page for my book on the CUP web site). A crucial step in understanding came with the realisation that, though the coefficients of inductance could be calculated in the same way, it is possible to infer the inductance from the capacitance since the phase velocity of TEM waves on the line is known. I realised that the same approach could be used for modelling helix slow-wave structures (the coefficients of capacitance can be calculated using WS 4.4).
A breakthrough came with the discovery that the properties of a structure with negligible dielectric loading could be modelled accurately if the capacitance and inductance of the sheath helix model were multiplied by constants that depended only on the dimensions of the structure. This breakthrough came through analysis of an unpublished set of careful experimental measurements of the properties of eleven structures made in 1996 by my Research Associate Dr P. Wang. The multiplying constant for the capacitance can be calculated using a 2D finite difference solution of Laplace’s equation when the phase shift between adjacent rings is zero. The constant for the inductance is then found by noting that the structure supports a helical TEM wave when the phase difference between adjacent turns of the helix is 180 degrees. The results obtained in this way agreed with measurements within the limits of experimental error (see pp. 162-165 and WS 4.5).
The method is readily extended to the case of a helix with dielectric support rods. If the rods are wedge-shaped then it is well-known they can be replaced by an equivalent uniform dielectric cylinder. The multiplying constant for the capacitance can be obtained using a 2D finite difference solution and the multiplying constant for the inductance is unchanged by the addition of dielectric loading. Thus the creation of the model requires only two finite difference calculations for each structure. If the rods are not wedge shaped it is still possible to represent them by an equivalent dielectric cylinder but with reduced accuracy. Ideally a 3D finite difference calculation should then be used to calculate multiplying constant for the capacitance. The use of a series of rings to represent the helix in these calculations does not seem to cause appreciable error.
Unfortunately, I was not able to confirm the validity of the method when dielectric rods were present because we had not taken sufficient care in our experiments to ensure that the rods were in contact with the helix in every case. I was able to show that the results of calculation agreed with the experimental results if a small air gap existed between the helix and the dielectric (which is easily modelled in the FD calculation). I also found that good agreement with the data for the impedance was obtained when the multiplying constant for the capacitance was chosen to give a good fit to the data for the phase velocity. This tends to confirm that the method is correct provided that the constant for the capacitance can be calculated for the experimental structure. The example reported in the book was obtained using published data for a structure with rectangular support rods. Further work is needed to confirm the accuracy of the method for structures with dielectric support rods of different shapes and with modification of the surrounding metal shield to change the dispersion characteristics.
Overall I believe that this work represents a significant step in the modelling of helix slow-wave structures because, for the first time, it avoids the need for a complex analytical solution and it allows the finite thickness of the helix tape to be included without any simplifying assumptions. Although two separate worksheets were used for the FD and the sheath helix models it would be straightforward to combine them into a single sheet so that the properties of a structure could be computed directly from its dimensions.
Read more about this in Chapter 4 Slow-Wave Structures available to read for free on Cambridge Core until 18 November 2019
Latest Comments
Have your say!